The Right Triangles

Pythagoras

When I was about eleven years old, my father grabbed a spool of string, some garden stakes, and his toolbox. The two of us walked outside, where he handed me the tools and said, “Alright, build me an outline of a rectangular flower bed that has right angles where any walls would meet.” Nothing quite says “I love you” like having your offspring draw right angles in the dirt.

Well, I looked at the tools and picked out the hammer and the speed square, which is essentially a set of three rulers having an identity crisis in the shape of a right triangle. I drove one stake into the ground (which was quite an ungainly procedure for such an uncoordinated child), wrapped it with string, and trotted off some distance to drive another stake into the ground. After tying the string to the second stake, I had a straight line. Perfect so far. I placed the speed square on the ground and lined one edge up to the string already in place. Following the right angle shown by the speed square, I took up a third stake, and trailed the string along. When the third stake and string looked perfectly aligned with the speed square, I drove that one into the ground as well. I repeated this process until I had all four sides of my father’s commissioned flower bed.

For the most part, the flower bed looked pretty square. My father pulled out a measuring tape and decided to check my work. Everyone knows that a rectangle should have two sides that are equal, and then two more that are also equal. Of course, all four could be equal, and that would be a square. But my garden plan didn’t have two sets of equal sides;Each leg was a different length, like how each year of high school felt progressively longer until senior year lasted approximately three decades.

I scurried around, shuffling the stakes, trying to get equal sides, but when I would get equal sides, I wouldn’t get right angles in the corners!

It was an impossible task, until my father suggested something rather genius: what if we drew out a length of string across the diagonal? Enter Pythagoras’s theorem - invented by a man whose cult was so obsessed with mathematics they allegedly drowned a member for discovering irrational numbers. Which, honestly, is still a more reasonable reaction than some of my high school classmates had to algebra. By using Pythagoras’s theorem of right triangles, we could ensure both the angles within the garden and the garden walls were properly set up.

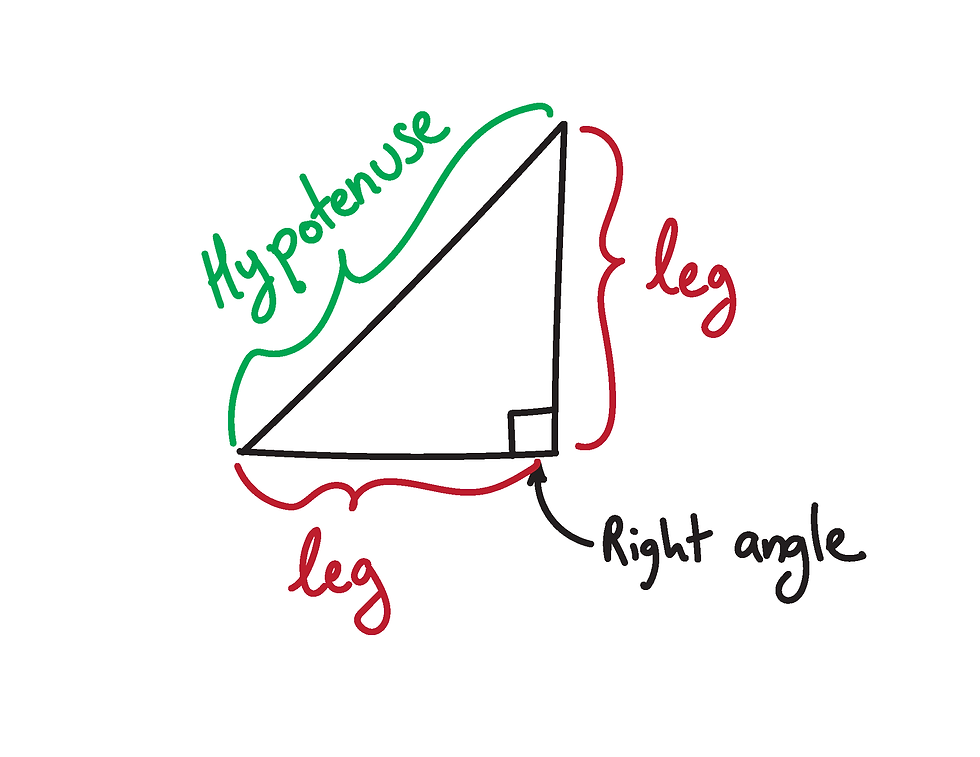
Pythagoras’s theorem of right triangles states that the hypotenuse of a right triangle is equal to the square root of the sum of each squared leg. As an equation, this means,

where c is the hypotenuse, and a, b are the legs of the triangle. This can be seen in Fig 1.

Figure 1: The anatomy of a right triangle. In this image, the side labeled “Hypotenuse” would correspond to Eq (1)’s c, and the sides labeled “leg” would correspond to Eq (1)’s a, b.

I can’t remember the exact size of the garden, but let’s just say I decided to make one side of the garden three feet long, and the other side to be four feet long. By putting these values into the Pythagorean theorem, I cut a piece of string that was five feet long, and shifted the sides so that the five-foot string touched the end of both sides. Because a rectangle can be made of two triangles, I simply had to do the same thing for the remaining two sides of the garden!

The lines were finally square and the length of the sides properly proportioned. The speed square agreed, and the measuring tape approved.

The issue with my first method was that the speed square was really excellent for determining the walls of a small garden, but after my string became several feet long, there was a good deal of wiggle room. Was it straight here? Was it too far to the left? I could move the string quite a lot but still believe it was square. With the Pythagorean theorem, however, there was only one way it would all work out; by having all the ends touch perfectly.

Trigonometry

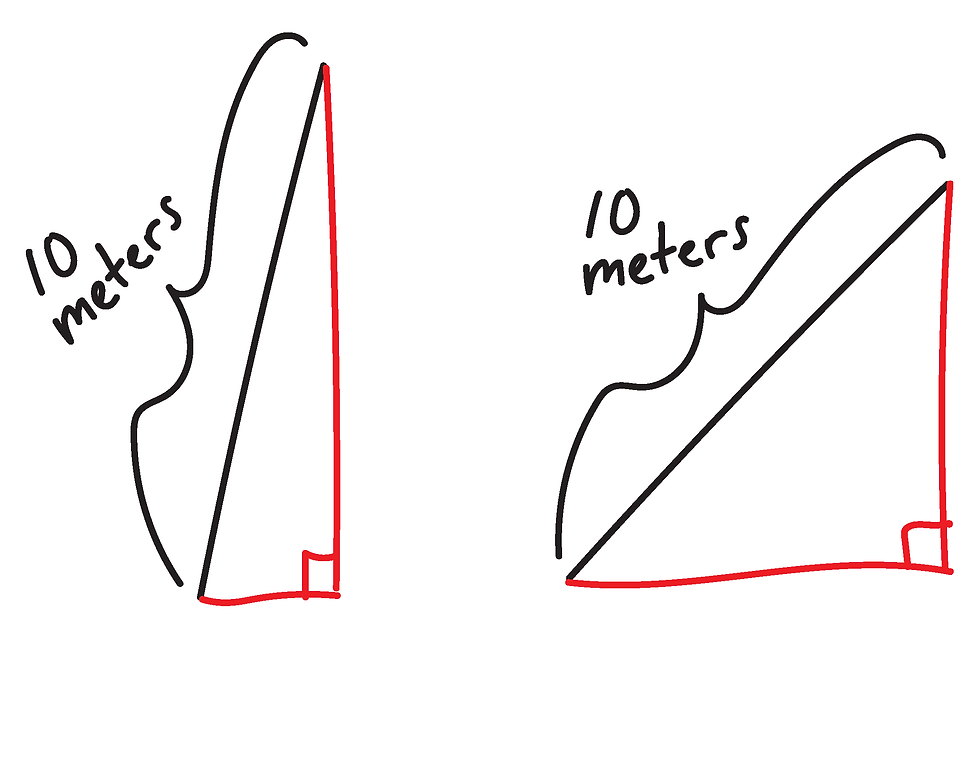
Now, if you know two sides of a triangle, using the pythagorean theorem is extremely useful. Sometimes, however, you don’t know two sides of a triangle. If you only know the length of one side of a triangle, there is simply no way for you to determine how large or small the other sides must be. For instance, if I know that the hypotenuse of a right triangle is 10 meters, I might draw either of the triangles in Fig 2.

Figure 2: Two obviously different triangles which have the same length of hypotenuse.

I can, however figure out all the lengths of all the sides of a triangle if I am given one side length, and one additional angle (apart from the right angle). We will call this angle θ (“theta”).

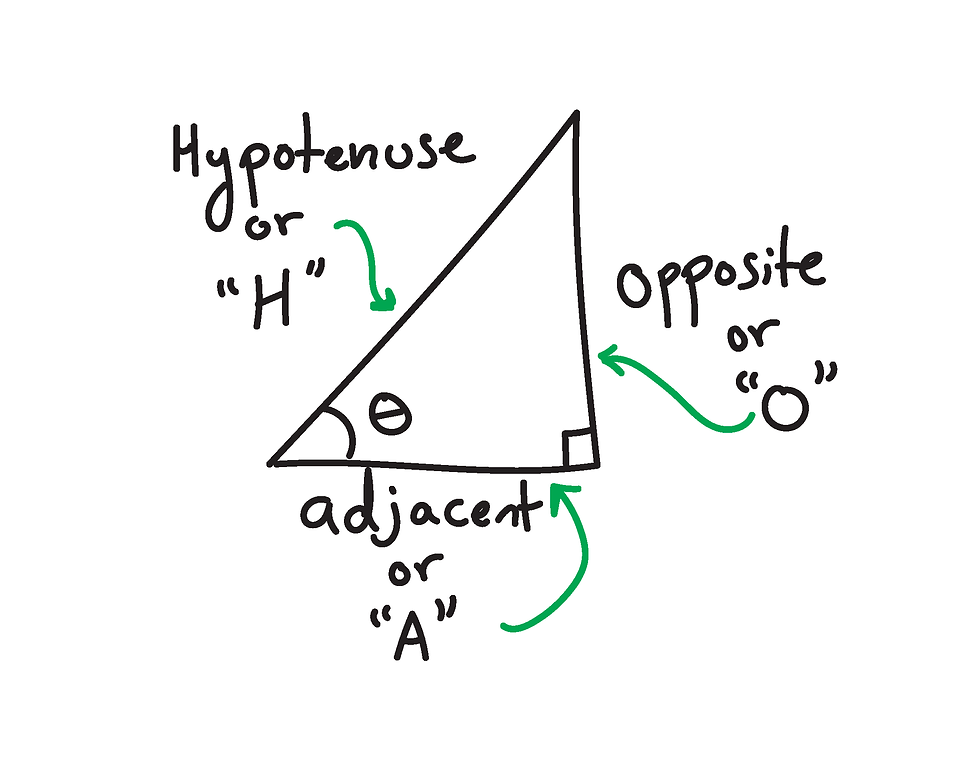
There are many mnemonics floating around, but the one I always remember is “SOH-CAH-TOA”. This is a compact way of saying “Sine of theta is equal to the opposite side over the hypotenuse, cosine of theta is equal to the adjacent side over the hypotenuse, and tangent of theta is equal to the opposite side over the adjacent side.” I suppose it is important to see that written out at least once, but it is much less confusing to simply write the equations,

and to observe the labeled triangle in Fig 3.

Figure 3: The labeled sides of a triangle for using the mnemonic SOH-CAH-TOA.

I might, for example, say that I have a hypotenuse of 10 meters, and an angle of

θ = π/3 . I can then figure out that the other sides of the triangle must be:

Figure 4: Finding the length of two sides of a triangle based on the hypotenuse and an angle.

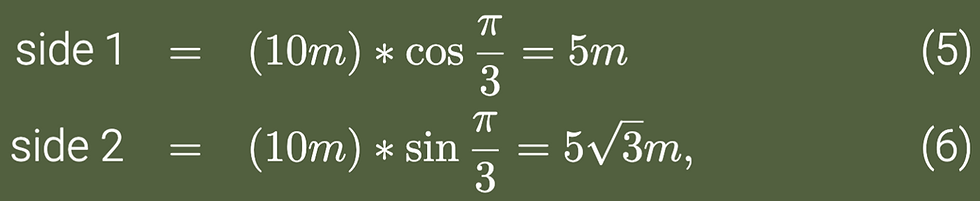
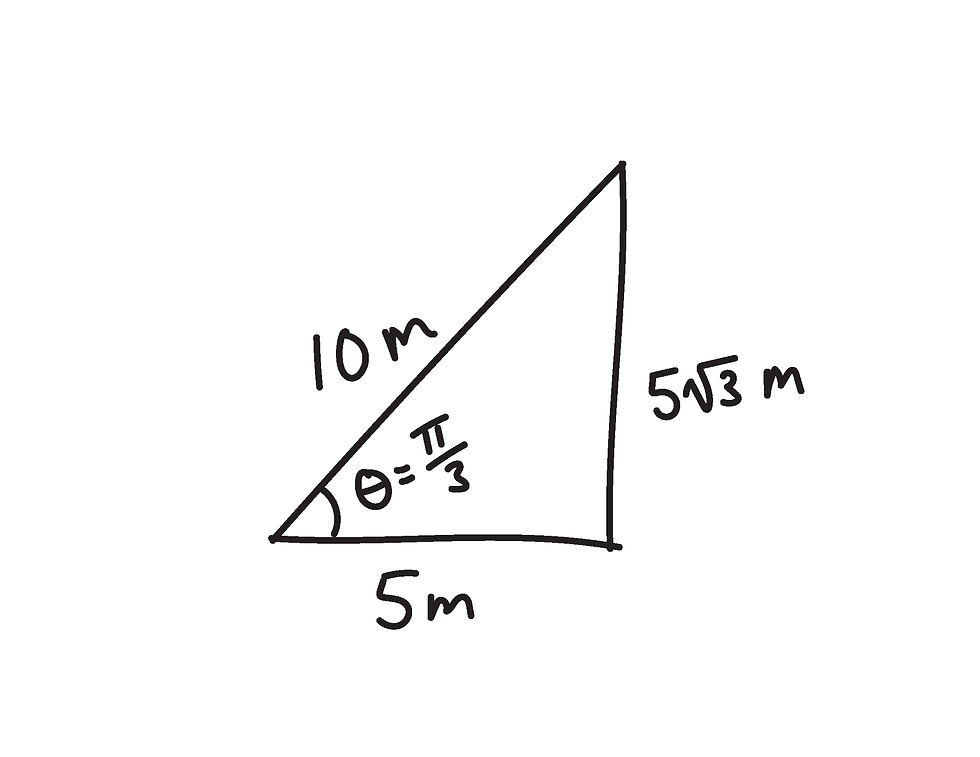
where side 1 makes the angle θ with the hypotenuse like in Fig 4.

The Caveat

Triangles are incredibly useful throughout physics, but there is a concern with all that I have said thus far; not all triangles bear the same foundational qualities. You may have come to define a triangle as a shape with three sides and internal angles that sum up to 180 degrees. This is absolutely correct if I draw a triangle on a piece of paper, but what if I draw a triangle on a globe? If I begin at the equator, and draw a straight line up to the North Pole, I have created a 90 degree angle with the equatorial line. If I then turn the globe a little bit and draw another line from the equator to the North Pole, I’ve created another 90 degree angle with the equatorial line. The two lines I drew plus the stretch of equator between the two lines combine to make a closed shape with three sides; a triangle. But it’s clear that 90 + 90+the angle at the North Pole is already greater than 180 degrees. So we have a triangle with angles that sum to more than 180 degrees. This is geometry’s way of saying ‘surprise! Everything is way more complicated than you imagined,’ which is basically a preview of your entire adult life.

So what we have thus far defined are right triangle rules which are good and true for Euclidean space, otherwise known as flat spacetime. They do not, however, work for curved spacetime.